Phonon-Polariton lasers

Theoretical model

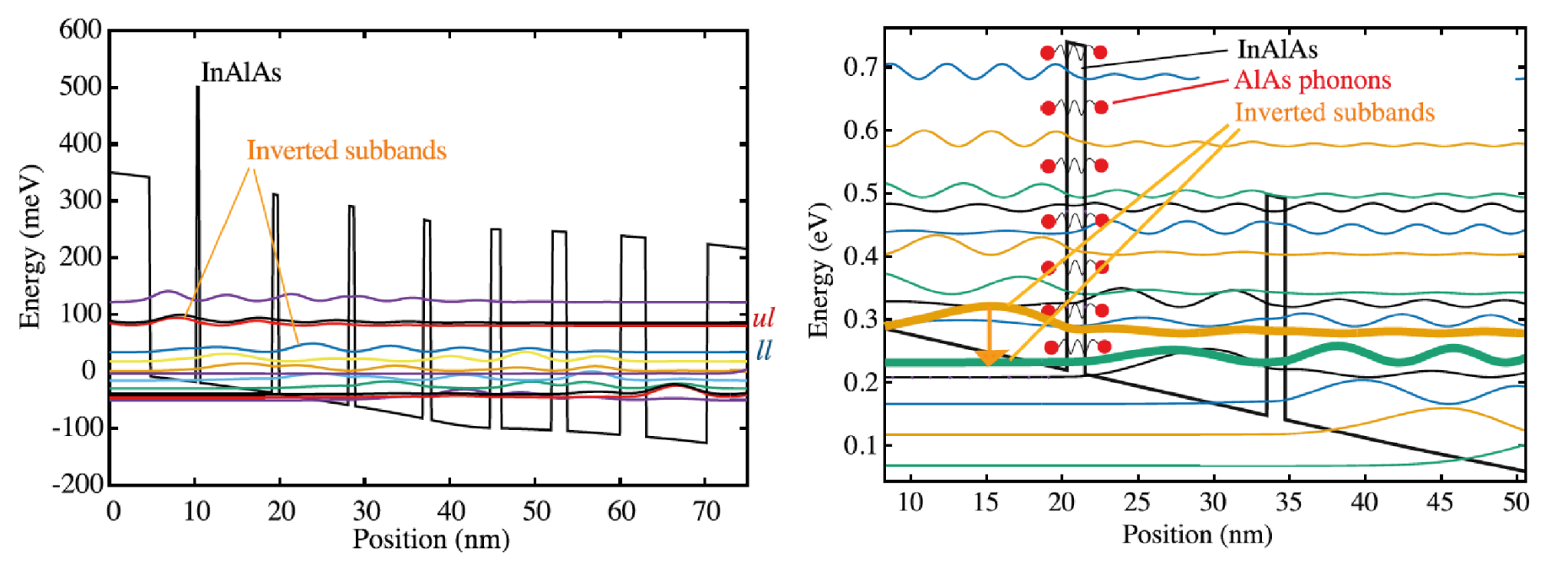
To describe this phenomenon and investigate ways to exploit it, we have developed a model for simulating the phonon polariton gain in a semiconductor heterostructure with population inversion, such as a quantum cascade laser (QCL) or resonant tunneling diode (RTD), shown in Fig. 2. [1]
 

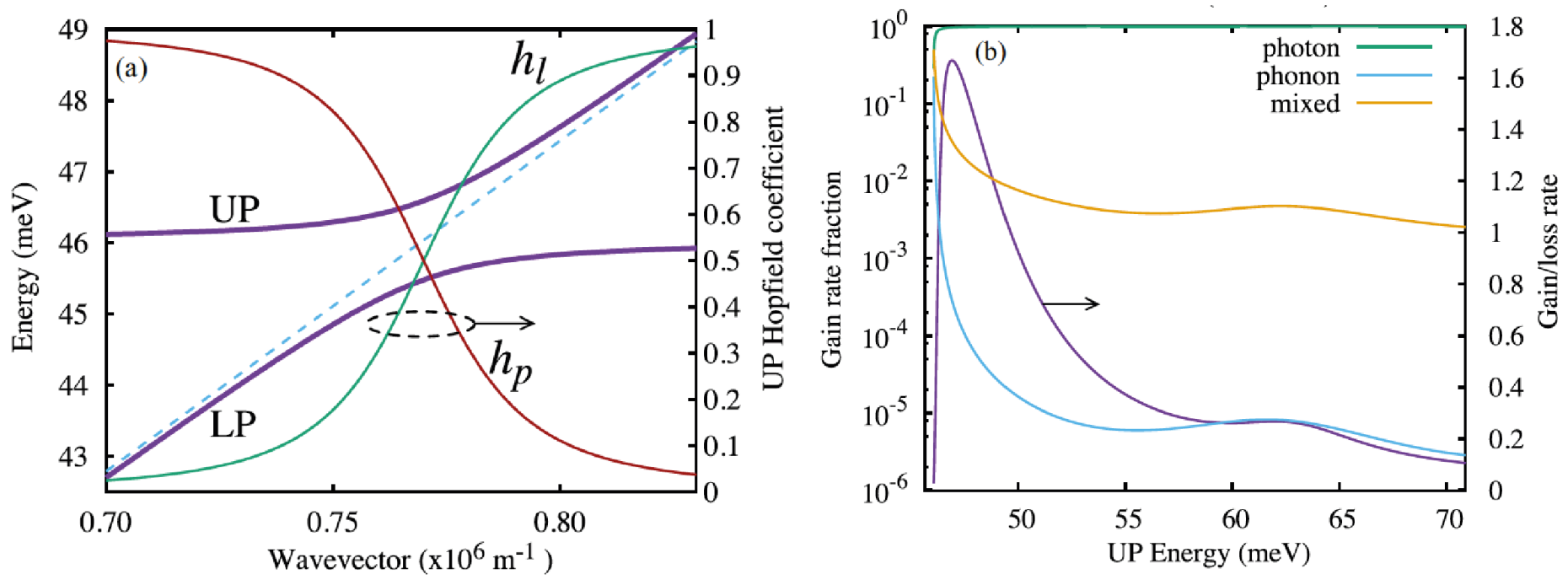
In these structures, the TO phonons which are in resonance with the laser transition are the AlAs-like phonon modes of the thin InAlAs barriers. Due to the strong light-phonon coupling, the straight photon dispersion line and the (amost) flat phonon dispersion line anti-cross at the resonant frequency and form two distinct polariton branches, as shown by thick purble lines in Fig. 3(a). The two branches are called “upper” (UP) and “lower” (LP) polariton branches, respectively. When going along the wave vector axis inside a particular polariton branch, the nature of the polariton alters between light-like to phonon-like at the two extremes, which is quantified by the Hopfield coefficients h_l and h_p.  

In Fig. 3(b) we instead focus on the energy axis in the UP branch, and model the gain rate of phonon polaritons for varying energy (right axis). We see that, over a large energy range it is possible to achieve a larger gain than the total loss (taking into account both phonon and photon losses). We also pay attention to the fraction of the gain coming from the phonon and photon parts, respectively (left axis). We note that while the gain rate is mostly originating from the photon part at high energy where the polariton is mostly photon-like, the gain close to the polariton gap (opening up for energies < 46 meV) is dominated by the phonon part for a short energy range. At the peak of the total gain rate, however, there is still a few percent non-photonic contribution. For this particular structure and emission frequency, the phonon loss rate (originating from decay into acoustic phonons) is actually lower than the photon one, and thus the laser operation is helped by the strong coupling to the phonons.

Experimental studies

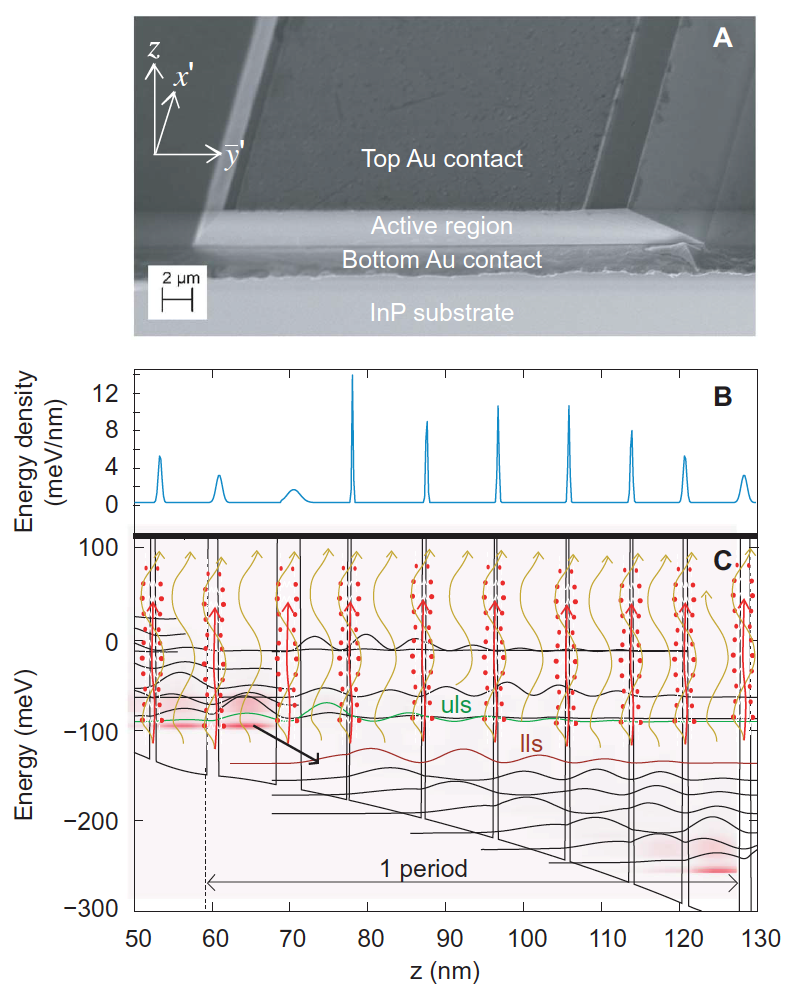
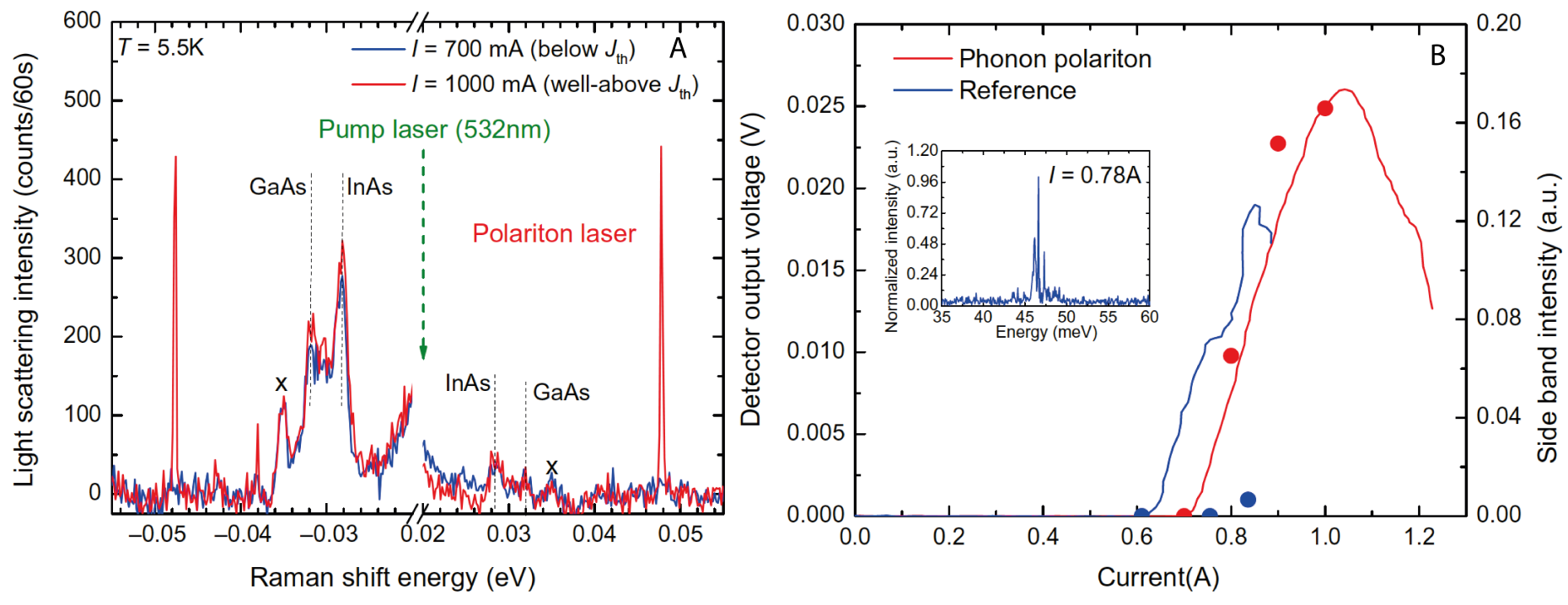
We have realized phonon-polariton lasers experimentally [2], shown in Fig. 4. In this design all barriers contain AlAs TO phonons. In order to measure the emitted phonon-polaritons, both the optical spectrum emitted from the laser, and the back-scattered Raman spectrum originating from excited phonon modes, has been measured. Fig. 5(a) shows the Raman spectra when the laser is below respectively above threshold. The narrow, tall peaks of equal height appearing at the expected phonon-polariton frequency above threshold, indicates that phonon-polaritons are coherently generated by stimulated emission in very large numbers. The optical spectrum features peaks at the same frequency, and both optical and phonon spectra show the same threshold behavior, as seen in Fig. 5(b). By tuning the frequency over a broad range for several devices with slight variations in designed emission frequency, we could deduce the group refractive index and match it to the calculated phonon-polariton dispersion as shown in Fig. 6.